I have already answered this question. It was posted. Then, it disappeared into the stratosphere. That's strange.
In any case, usually, this expression would be in its simplified form already. However, in this case, the radicands (numbers inside of the radical) happen to be perfect squares, so this expression can be simplified further:
| \(2\sqrt{4}+5\sqrt{9}\) | \(\sqrt{4}=2\hspace{1mm}\text{and}\hspace{1mm}\sqrt{9}=3\) |
| \(2*2+5*3\) | Now, it is a matter of simplifying from here. |
| \(4+15\) | |
| \(19\) | |
No, the expression evaluates to 39:
| \(3\left[\frac{30-8}{2}+2\right]\) | Do 30-8 first. |
| \(3\left[\frac{22}{2}+2\right]\) | Do 22/2 next. Luckily, 2 divides into 22 evenly. |
| \(3\left[11+2\right]\) | Do 11+2 after that. |
| \(3[13]=3*13=39\) | |
I'll help you simplfy this expression \(\left(\frac{1}{2}\right)^2-6\left(2-\frac{2}{3}\right)\):
| \(\left(\frac{1}{2}\right)^2-6\left(2-\frac{2}{3}\right)\) | To abide to order of operations, I will start with the parentheses 2-(2/3). |
| \(\frac{2}{1}-\frac{2}{3}\) | In order to subtract fractions, we must establish a common denominator. Currently, this isn't the case. To do that, we must figure out the LCM (lowest common multiple) of the denominators. In this case, one of the denominators is 1. That means that the LCM is whatever the other number is. The LCM is 3. Let's convert the fraction |
| \(\frac{2}{1}*\frac{3}{3}=\frac{6}{3}\) | Note that I am really multiplying the fraction by 1, so I am not changing the value of the fraction. |
| \(\frac{6}{3}-\frac{2}{3}=\frac{4}{3}\) | Of course, when subtraction fractions, you only subtract the numerator. |
| \(\left(\frac{1}{2}\right)^2-6*\frac{4}{3}\) | Yet again, to abide by the rules of the order of operations, I will now do the exponent. I will apply the rule that \(\left(\frac{a}{b}\right)^n=\frac{a^n}{b^n}\) |
| \(\left(\frac{1}{2}\right)^2=\frac{1^2}{2^2}=\frac{1}{4}\) | I just applied the rule that I mentioned before. |
| \(\frac{1}{4}-6*\frac{4}{3}\) | Now, I will do the multiplication of 6*(4/3) |
| \(\frac{6}{1}*\frac{4}{3}=\frac{24}{3}=8\) | This is the multiplication done now. Now, we can move on to the next step. |
| \(\frac{1}{4}-8\) | Yet again, manipulate 8 such that there are common denominators. |
| \(\frac{8}{1}*\frac{4}{4}=\frac{32}{4}\) | |
| \(\frac{1}{4}-\frac{32}{4}\) | Do the subtraction. |
| \(-\frac{31}{4}=-7\frac{3}{4}\) | |
Therefore, \(\left(\frac{1}{2}\right)^2-6\left(2-\frac{2}{3}\right)=-\frac{31}{4}=-7\frac{3}{4}\)
.Here is a picture of your diagram:
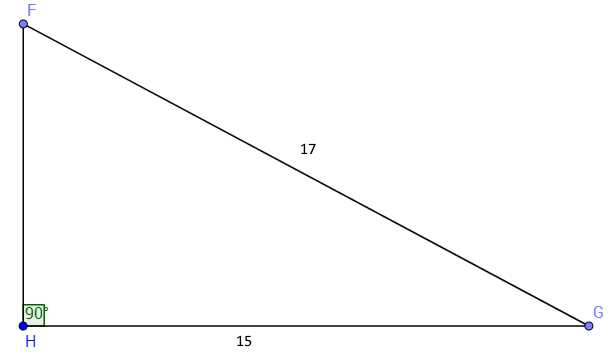
In this diagram, I have labeled all of the given info on it. Our goal is to figure out what \(\sin\angle G\) is. In right-triangle trigonometry, the sine function compares, from the angle of reference, the opposite angle to the hypotenuse. Let's do that:
| \(\frac{\sin m\angle G}{1}=\frac{FH}{17}\) | Uh oh! We don't know what the length of the segment of FH. We can use Pythagorean theorem. If you are like me, I have memorized some Pythagorean triples (integer solutions to the Pythagorean theorem like 3,4, and 5), but I will solve for them anyway. |
Let's apply the Pythagorean Theorem to figure out the remaining side:
| \(FH^2+15^2=17^2\) | Simplify both sides of the equation before continuing. |
| \(FH^2+225=289\) | Subtract 225 from both sides. |
| \(FH^2=64\) | Take the square root of both sides to undo the exponent of 2. |
| \(|FH|=8\) | Of course, the absolute value splits the possible answers to its positive and negative form. |
| \(FH=\pm8\) | Of course, in the context of geometry, a negative side length is nonsensical, so we will should reject the negative answer. |
| \(FH=8\) | |
Now that we know what FH is, we can plug it back into what we were originally solving for, \(\sin m\angle G\):
| \(\sin m\angle G=\frac{8}{17}\) | Technically, I should stop here, as the question asked for the value of \(\sin m\angle G\), but I will solve for G just in case that is actually what you are solving for. To solve for the angle measure, you must use the inverse function. |
| \(\sin^{-1}\left(\frac{8}{17}\right)=m\angle G\) | Use a scientific calculator with trigonometric functions to approximate the measure of the angle. Since an angle is measured in degrees, the calculator should be in degree mode when the expression is inputted. |
| \(m\angle G=\sin^{-1}\left(\frac{8}{17}\right)\approx28.07^{\circ}\) | |
We're done now!
We want to solve for b in the equation \(-\frac{3}{4}b=2\). Let's do that!
| \(-\frac{3}{4}b=2\) | Multiply both sides of the equation by 4 to get rid of the denominator. |
| \(-3b=8\) | Divide both sides by -3 |
| \(b=-\frac{8}{3}=-2\frac{2}{3}=-2.\overline{666}\) | |
Let's think about this problem. There are 2 solutions to \(y!=1\). It is 0 and 1. Therefore, we need to set \(3x-2\) equal to both 0 and 1:
| \(3x-2=0\) | Add 2 to both sides. |
| \(3x=2\) | Divide by 3 on both sides. |
| \(x=\frac{2}{3}\) | |
Let's do the other one now!
| \(3x-2=1\) | Add 2 to both sides. |
| \(3x=3\) | Divide by 3 on both sides. |
| \(x=1\) | |
Therefore, x=1 and 2/3
To do \(\left(\frac{1}{2}-\frac{2}{5}\right)^2\), we will first have to convert both fractions into fractions that have common denominators. To do this, we must figure out the LCM (lowest common multiple) of both denominators in the expression. In this example, 2 and 5 are co-prime, so simply multiply them together to figure out the LCM. The LCM is 2*%, or 10. Let's convert both fractions such that both have a denominator of 10. I will manipulate the fractions chronologically in which they appear in the expression:
| \(\frac{1}{2}*\frac{5}{5}\) | Note that we are really multiplying the fraction by 1, so the actual value of the fraction will be unchanged. |
| \(\frac{5}{10}\) | |
And of course, the next fraction:
| \(\frac{2}{5}*\frac{2}{2}\) | This accomplishes the exact same thing as above--getting a common denominator. |
| \(\frac{4}{10}\) | |
Let's subtract the fractions, now:
| \(\left(\frac{5}{10}-\frac{4}{10}\right)^2\) | Subtracting the fraction involves subtracting the numerator and keeping the denominator unchanged. |
| \(\left(\frac{1}{10}\right)^2\) | To square a fraction, use the following rule of \(\left(\frac{a}{b}\right)^n=\frac{a^n}{b^n}\). You are "distributing" the fraction to both the numerator and denominator. |
| \(\frac{1^2}{10^2}\) | Simplify both the numerator and denominator. |
| \(\frac{1}{100}\) |
|
Therefore, \(\left(\frac{1}{2}-\frac{2}{5}\right)^2=\frac{1}{100}\)
.I am coming to help! You want to know how to evaluate the expression \(\frac{4}{5}-\frac{1}{6}\):
To subtract (or add) fractions, we have to create a common denominator. What does that mean? It means that the denominators of both fractions must be the same before we can actually do any subtraction. 4/5 and 1/6 are not fractions with common denominators, so we will have to manipulate the fraction such that both have a common denominator.
To do this, we must figure out the LCM (lowest common multiple) of both denominators in this expression. Normally, you could use a process to figure out the lowest common denominator, but we can actually use a shortcut here. If you are trying to find the LCM of two numbers that is 1 unit away from each other on a number line, then simply multiply both numbers together. This means that the LCM of 5 and 6 is 5*6, or 30:
This is the denominator that we want both fractions to have. Let's manipulate 4/5 first:
| \(\frac{4}{5}*\frac{6}{6}\) | Notice that we are actually multiplying the fraction by 1, which does not change the actual value of the fraction. |
| \(\frac{24}{30}\) |
|
Now, let's change the other fraction such that the denominator is 30:
| \(\frac{1}{6}*\frac{5}{5}\) | Just like above, I am making the denominator to 30. |
| \(\frac{5}{30}\) |
Now, let's subtracting the 2 fractions! It should be much easier now!
| \(\frac{24}{30}-\frac{5}{30}\) | When subtracting fractions, only subtract the numerator, but keep the denominator. |
| \(\frac{19}{30}\) | 19 and 30 are co-prime, so the fraction cannot be simplified anymore. |
Therefore, \(\frac{4}{5}-\frac{1}{6}=\frac{19}{30}\)
.