Apologies, I am jumping at this problem with only knowledge up to High School Geometry Right Triangle Trigonometry Unit (yes, I am an uneducated idot, deal with it.)
According to my inaccurate intuition, if he swam at an angle, his path will be circular (or elliptical?). I am to proceed with this inaccurate intuition.
I drew the following image on Scratch. (Yes, my hobby is making video games then getting addicted to my own video games)
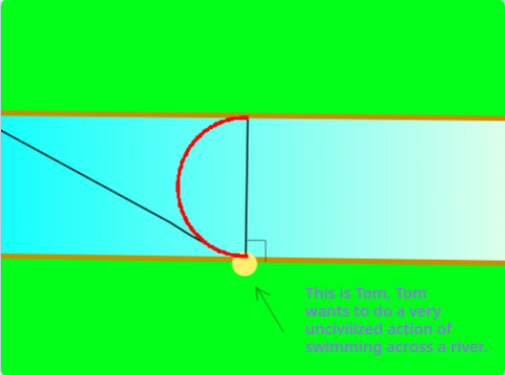
So the angle he swims at shall be tangent to that semi-circular path? I think my intuition serves me incorrectly.
At this point, I realize my intuition was unreliable and I decided to look up the answer on Chegg. Unfortunately, I do not own a Chegg account, so I refused the $20 monthly fee as confronting my parents to give me 20 bucks a month to help someone online with a math problem wouldn't be a wise decision.
Again using my amazing intuition, I imagine Tom swimming straight forward at 2km/h with the river pushing him at 1km/h.
According to my intuition, he will have moved down the river 250 meters when he crossed the 500 meter wide river.
Alright, then using my intuition once again, the angle in which he must swim at to offset that 250 meters is basically the angle formed by a triangle whose legs are in a 1:2 ratio.
So by finally using something else then my intuition, I used a calculator (hence my name) and calculated for this:
\(tan^{-1}(\frac{1}{2})\)
asin(1/2) = 26.565051177078
So I got 90 - 26.565051177078 = WHATEVER THIS IS
In other words, I probably learn from someone else's answer if I am incorrect.