Dragan

In parallelogram the adjacent angles are supplementary.

Angle  A = 62°         

Angle  ADC = 180° - 62° = 118°   

They probably meant this  ( see diagram )  

r = 27.8

Area of sector   A_s = [(r²pi) /360°] * 150° = 1011.645194 in²

Area of triangle   A_t = [ sin(15°) * 27.8] * [ cos(15°) * 27.8 ] = 193.21 in²

Shaded area       A = A_s - A_t = 818.435194 in²   

5 Faces        5 Vertices      Edges = ?

F + V = E + 2

E = F + V - 2             E = 8    

That was so difficult 

Select the correct answer.

If quadrilateral ABCD rotates 90° counterclockwise about the origin, what are the coordinates of A′ in quadrilateral A′B′C′D′ ?

A' ( -2, -1 )

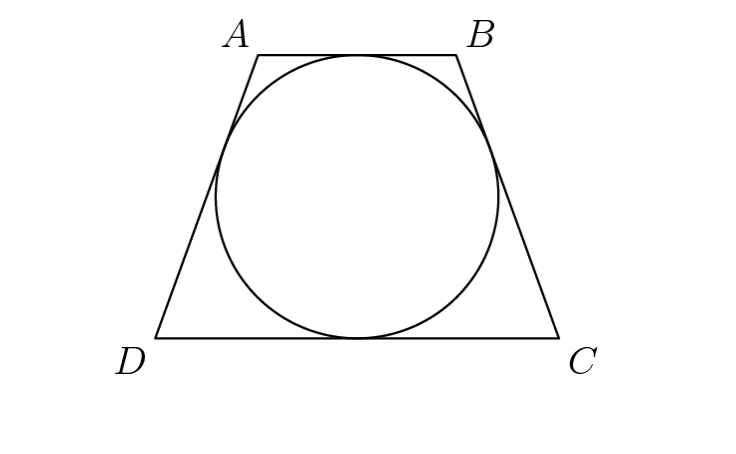
Let ABCD be an isosceles trapezoid, with bases AB and CD. and  A circle is inscribed in the trapezoid, as shown below. (In other words, the circle is tangent to all the sides of the trapezoid.) The length of base AB is 2x and the length of base CD is 2y. Prove that the radius of the inscribed circle is sqrt(xy).

I'll use the real numbers:

AB = 2x = 5    x = 2.5         CD = 2y = 10     y = 5

AD = BC = AB/2 + CD/2 = 7.5

Radius r = ?          r =  {sqrt [(AD)² - (CD/4)²]} /2  = 3.535533906

                                       sqrt (xy) = sqrt (2.5 * 5) = 3.535533906

If the arc of ABC is 244 degrees, what is the number of degrees in the measure of ABC? 

Angle   ABC = 360° - 244° = 116°  

A non-isosceles right triangle XYZ has the area of  1m squared. Find  the parameter.

This is interesting...

If the area of the right triangle is 1m², than one leg is 1m and the other leg is 2m

                                                               (1m * 2m) /2 = 1m²

Soooo,   the parameter(P) is:     P = 1 + 2 + sqrt(5) m   

This approach makes more sense to me. ( I watched the video )  

(Instead of  CD  I put  AB )    

Good job, Guest!!!