A square $DEFG$ varies inside equilateral triangle $ABC,$
so that $E$ always lies on side $\overline{AB},$ $F$ always lies on side $\overline{BC},$
and $G$ always lies on side $\overline{AC}.$
The point $D$ starts on side $\overline{AB},$ and ends on side $\overline{AC}.$
The diagram below shows the initial position of square $DEFG,$ an intermediate position, and the final position.

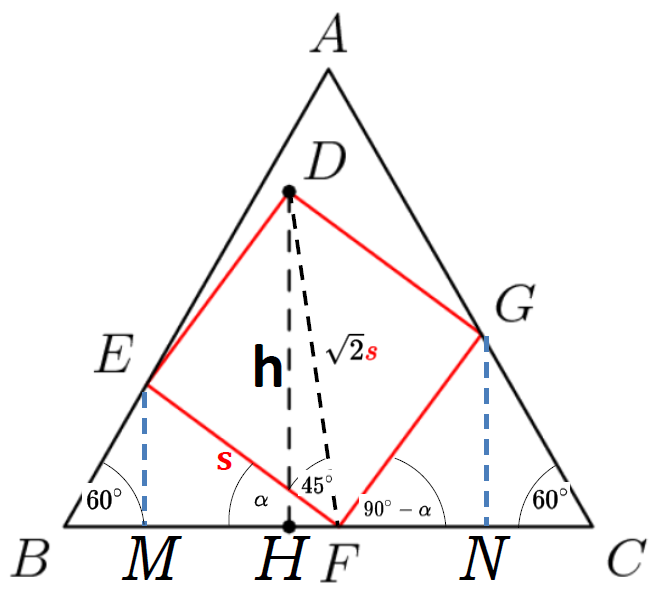
Show that as square DEFG varies, the height of point D above line BC remains constant.
Let ¯DH=h the height of point D above line ¯BC Let s the side of the square DEFG Let ¯DF=√2s Let ∠EFD=45∘ Let ∠BFE=α Let ∠CFG=90∘−α The equilateral triangle ABC has the angles 60∘, 60∘, 60∘ and the sides a, a, a.
In the rectangular triangle DHF the following applies:
sin(α+45∘)=h√2s√2sin(α+45∘)=hs|√2sin(α+45∘)=sin(α)+cos(α)sin(α)+cos(α)=hsh=ssin(α)+scos(α)
Let ¯BC=a is the side of the equilateral triangle ABC.
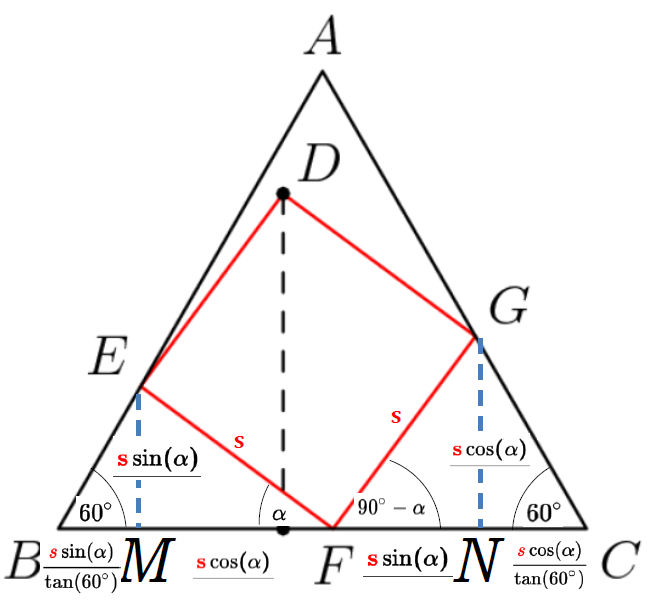
a=¯BM+¯MF+¯FN+¯NC¯BM=ssin(α)tan(60∘)¯MF=scos(α)¯FN=ssin(α)¯NC=scos(α)tan(60∘)a=ssin(α)tan(60∘)+scos(α)+ssin(α)+scos(α)tan(60∘)a=ssin(α)+scos(α)+(ssin(α)+scos(α))1tan(60∘)a=(ssin(α)+scos(α))(1+1tan(60∘))|h=ssin(α)+scos(α)a=h(1+1tan(60∘))|tan(60∘)=√3a=h(1+1√3)a=h(√3+1√3)h=a(√31+√3)
So the height of point D above line BC remains constant.
