Two 2*8 rectangles overlap, as shown below.
Find the area of the overlapping region (which is shaded)
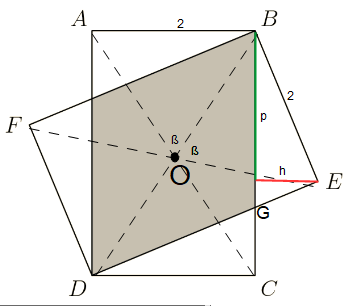
\(\text{Let $O=(0,0)$} \\ \text{Let $A=(-1,4)$} \\ \text{Let $B=(1,4)$} \\ \\ \text{Let $E=(x_E,y_E)$} \\ \text{Let area of rectangle $=2*8$ } \\ \text{Let area of triangle $[BEG]=\dfrac{\overline{BG}*h}{2}$ } \\ \text{Let the gray area $=$ area of rectangle$-2*$ area of triangle $[BEG]$ } \\ \text{Let the gray area $= \mathbf{2*8-\overline{BG}*h}$ } \\ \text{Let $h=x_E-x_B$} \\ \text{Let $p=y_B-y_E$} \\ \text{Let $\overline{AB}=\overline{BE}=2$} \\ \text{Let $\overline{BE}^2=p*\overline{BG}$} \)
1. rotate
\(\begin{array}{|lrcll|} \hline \text{Rotation matrix } \\ & R&=&\begin{bmatrix} \cos(ß)&\sin(ß) \\ - \sin(ß)&\cos(ß) \\ \end{bmatrix} \\ & B&=&R\cdot A \\\\ & \begin{bmatrix} x_B\\y_B\end{bmatrix} &=&\begin{bmatrix} \cos(ß)&\sin(ß) \\ - \sin(ß)&\cos(ß) \\ \end{bmatrix} \begin{bmatrix} x_A\\y_A\end{bmatrix} \\ \hline \end{array}\)
\(\begin{array}{|rcll|} \hline \begin{bmatrix} 1\\4\end{bmatrix} &=&\begin{bmatrix} \cos(ß)&\sin(ß) \\ - \sin(ß)&\cos(ß) \\ \end{bmatrix} \begin{bmatrix} -1\\4\end{bmatrix} \\\\ -\cos{ß}+4\sin(ß) &=& 1 \qquad (1) \\ \sin{ß}+4\cos(ß) &=& 4 \qquad (2) \\ \\ \mathbf{\sin(ß)} &=& \mathbf{\dfrac{8}{17}} \\\\ \mathbf{\cos(ß)} &=& \mathbf{\dfrac{15}{17}} \\ \hline \end{array}\)
2. rotate
\(\begin{array}{|rcll|} \hline \begin{bmatrix} x_E\\y_E\end{bmatrix} &=&\begin{bmatrix} \cos(ß)&\sin(ß) \\ - \sin(ß)&\cos(ß) \\ \end{bmatrix} \begin{bmatrix} 1\\4\end{bmatrix} \\\\ \cos{ß}+4\sin(ß) &=& x_E \qquad (3) \\ x_E &=& \dfrac{15}{17} + 4*\dfrac{8}{17} \\ \mathbf{x_E} &=& \mathbf{\dfrac{47}{17}} \\\\ -\sin{ß}+4\cos(ß) &=& y_E \qquad (4) \\ y_E &=& \dfrac{8}{17} + 4*\dfrac{15}{17} \\ \mathbf{y_E} &=& \mathbf{\dfrac{52}{17}} \\ \hline \end{array}\)
\(\begin{array}{|rcll|} \hline \mathbf{h}&=&\mathbf{x_E-x_B} \\\\ h&=&\dfrac{47}{17}-1 \\\\ \mathbf{h}&=&\mathbf{\dfrac{30}{17}} \\\\ \hline \mathbf{p} &=& \mathbf{y_B-y_E} \\\\ p &=& 4- \dfrac{52}{17} \\\\ \mathbf{p}&=&\mathbf{\dfrac{16}{17}} \\\\ \hline \mathbf{\overline{BE}^2} &=& \mathbf{p*\overline{BG}} \quad | \quad BE=2 \\ 2^2 &=& \dfrac{16}{17}*\overline{BG} \\ \overline{BG} &=& \dfrac{4}{\dfrac{16}{17}} \\\\ \mathbf{\overline{BG}} &=& \mathbf{\dfrac{17}{4}} \\\\ \hline \mathbf{\text{Gray area }} &=& \mathbf{2*8-\overline{BG}*h} \\ &=& \mathbf{2*8-\dfrac{17}{4}*\dfrac{30}{17}} \\\\ &=& 2*8-\dfrac{30}{4} \\\\ &=& 16-\dfrac{15}{2} \\\\ &=& \dfrac{32-15}{2} \\\\ &=& \dfrac{17}{2} \\\\ \mathbf{\text{Gray area }} &=& \mathbf{8.5} \\ \hline \end{array}\)
