See the following image :
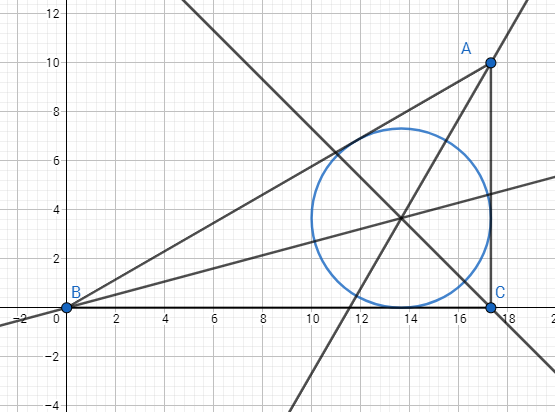
The center of the incircle will be the intersection of the angle bisectors shown
And the find the x coordinate of the center by solving these two equations :
y = tan (135) [x -10sqrt(3)] and y = tan(60) [x - 10sqrt (3)] + 10
Set these equations equal and we have
tan (135) [x -10sqrt(3)] = tan(60) [x - 10sqrt (3)] + 10 simplify
-1 [x - 10sqrt (3) ] = sqrt (3) [ x - 10sqrt (3) ] + 10
10sqrt (3) - x = sqrt (3) x - 30 + 10
x ( sqrt (3) + 1 ) = 10sqrt (3) + 20
x ( sqrt (3) + 1) = 10 [ sqrt (3) + 2 ]
x = 10 [ sqrt (3) + 2 ] / [ sqrt (3) + 1 ] multiply top /bottom by sqrt (3) - 1 and we have
x = 10 [ sqrt (3) + 2 ] [ sqrt (3) - 1 ] / [ 3 - 1]
x = 10 [ sqrt (3) + 2 ] [ sqrt (3) - 1 ] / [ 2]
x = 5 [ 3 + sqrt (3) - 2]
x = 5 [ 1 + sqrt (3) ] = 5 + 5sqrt (3)
And the y coordinate of the center is :
And y = -1 [ 5 + 5sqrt (3) - 10sqrt (3) ] = -1 [ 5 - 5sqrt (3) ] = 5sqrt (3) - 5 =
5 [ sqrt (3) - 1 ] and this is the radius of the incircle


