Note that the area of the first equilateral triangle is just (1/2)a^2 sin (60°)
And the area of the second equilateral triangle is just (1/2)b^2sin(60°)
So....the sum of their areas is just
(1/2) sin (60°) (a^2 + b^2)
So....we actually need the side of our constructed triangle to be √[a^2 + b^2]
Here's a way to do this ....a pic will definitely help !!!!
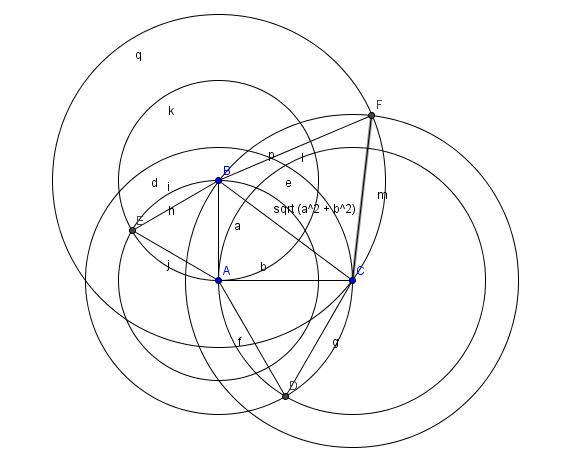
1. Draw any segment AC and then constuct any segment AB perpendicular to this....Connect BC...call AB, "a" and AC, "b" and BC = sqrt (a^2 + b^2)
2. Put your compass at A and draw a circle with radius AC.....then put it at C and draw a circle with radius CA.....let these circles intersect at "D" and connect AD and CD....this will create equilateral triangle ADC
3. Similarly......put your compass at A and draw a circle with radius AB....then put your compass at B and draw a circle with radius BA...let these intersect at E....this will create equilateral triangle BEA
4. Finally.....put your compass at C and and draw a circle with radius CB......then put your compass at B and draw a circle with radius BC.....let these circles intersect at F....this will create equilateral triangle CFB
So.....area of ADC = (1/2) a^2 sin (60°) and area of BEA = (1/2) b^2 sin (60°)
So their combined area = (1/2) sin (60°) (a^2 + b^2)
And the area of CFB = (1/2)sin (60°)√[a^2 + b^2] * √ [a^2 + b^2] =
(1/2) sin(60°) (a^2 + b^2)..... the combined areas of ADC and BEA !!!
Here's a pic without the circles:



