Suppose we have a square
with vertices at \((0),~ (-6+13i),~ (-19+7i) ,~~\text{and}~ (-13-6i)\).
Suppose that we want to multiply these points
by a single complex number a+bi to get a square
with vertices \((0),~ (8+2i),~ (6+10i),~ ~\text{and}~ (-2+8i)\).
What is (a,b)?
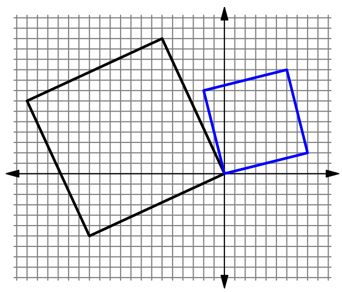
I assume:
We have a black square with vertices: \(\mathbf{z =|z|e^{i\varphi}}\)
We have a blue square with vertices: \(\mathbf{w =|w|e^{i\omega}}\)
We have a single complex number: \(\mathbf{x=a+bi}\)
Formula: \(\mathbf{w = x\cdot z \qquad \text{with}\qquad x = \dfrac{|w|}{|z|}e^{i(\omega-\varphi)} }\)
\(\text{For example we set $z=(-6+13i) \quad \text{and} \quad w=(8+2i)$ }\)
\(\begin{array}{|rcll|} \hline |z| &=& \sqrt{ \left(-6\right)^2+13^2 } \\ &=& \sqrt{36+169} \\ &=& \sqrt{205} \\ \mathbf{|z|} &=& \mathbf{14.3178210633}\\\\ |w| &=& \sqrt{8^2+2^2} \\ &=& \sqrt{68} \\ \mathbf{|w|} &=& \mathbf{8.2462112512}\\\\ \dfrac{|w|}{|z|}&=& \dfrac{8.2462112512}{14.3178210633} \\\\ \mathbf{\dfrac{|w|}{|z|}}&=& \mathbf{0.5759403763} \\ \hline \end{array}\)
\(\begin{array}{|rcll|} \hline \omega-\varphi &=& \arctan\left(\dfrac{2}{8}\right) -\arctan\left(\dfrac{13}{-6}\right) \\ &=& 14.03624346791^\circ - (180^\circ-65.2248594312^\circ) \\ &=& 14.03624346791^\circ - 114.775140569^\circ \\ \mathbf{\omega-\varphi} &=& \mathbf{-100.738897101^\circ} \\ \hline \end{array}\)
\(\begin{array}{|rcll|} \hline \mathbf{x} &=& \mathbf{\dfrac{|w|}{|z|}*e^{i(\omega-\varphi)}} \\\\ x &=& 0.5759403763*e^{i(-100.738897101^\circ)} \\\\ x &=& 0.5759403763* \Big( \cos(-100.738897101^\circ)+i*\sin(-100.738897101^\circ) \Big) \\ x &=& 0.5759403763*(-0.1863336512-0.9824865243i) \\ x &=&-0.1073170732-0.5658536585i \\\\ \mathbf{a} &=& \mathbf{-0.1073170732} \\ \mathbf{b} &=& \mathbf{-0.5658536585} \\ \hline \end{array}\)
\((a,~b) = (-0.1073170732,~-0.5658536585)\)
